Scientists often use ultracold atoms to study the behavior of atoms and electrons in solids and liquids (a.k.a. condensed matter). Their goal is to uncover microscopic quantum behavior of these condensed matter systems and develop a controlled environment to model materials with new and advanced functionality.
In an exciting new theory investigation, Fellow Ana Maria Rey and research associate Leonid Isaev have showed how ultracold atoms in optical lattices (created with intersecting laser beams) can model the interplay of two fundamental factors affecting the flow of electrons in metals: (1) localized magnetic impurities that behave as tiny magnets and (2) quantum frustration that occurs when the spins of the impurities cannot decide whether to point up or down.
When mobile electrons collide with impurities, their flow that generates a current is distorted, similarly to how obstacles distort waves in the sea. This process is how ordinary metals acquire resistance. However, when the impurities are magnetic and have a permanent magnetic moment, or spin, there is an additional contribution to resistance due to electron collisions accompanied by spin flips. (This behavior can occur because electrons have their own spin. Interactions between two electrons with spin-up/spin-down, respectively, can cause each one's spin orientation to change, or flip, to the opposite direction, i.e., to spin-down/spin-up.) This purely quantum effect profoundly modifies the properties of a metal. For instance, to an outside observer, mobile electrons appear a thousand times heavier than they really are because they tend to spend more time talking to the local spins and hence move slower.
Naturally, the spin-flipping collisions of electrons depend on how easily the electrons can modify the state of magnetic impurities. If the local spins are rigid and cannot be flipped, the system behaves as an ordinary metal. An especially interesting situation occurs when this rigidity arises because of competing spin-spin interactions with other nearby impurities. Whenever these interactions occur, the magnet is said to be frustrated because a magnetic impurity does not know if it should allow the mobile electron to flip its spin, or if instead it should remain rigid, obeying its surrounding localized partners. As a result of this confusion, the individual spins experience wild quantum fluctuations. Clearly, magnetic frustration should prevent electrons from efficiently communicating to the local spins, hence making them lighter. But figuring out which tendency wins remains a puzzle for scientists.
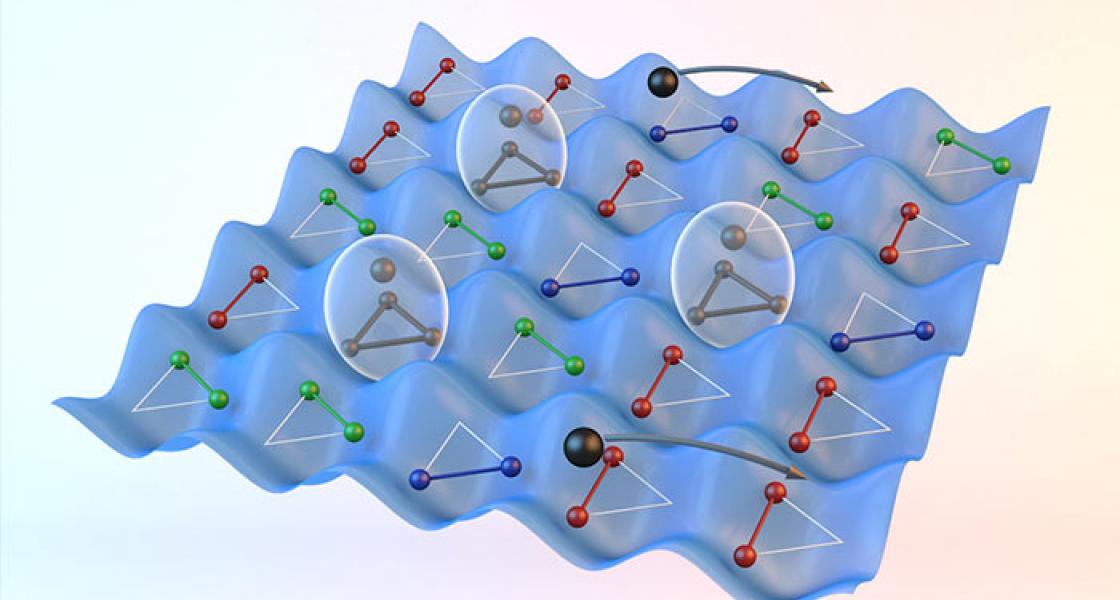
Interestingly, observing ultracold alkaline-earth atoms in an optical lattice can shed some light on this issue. The ultracold atoms can move and their nuclei have spins: the former allows scientists to model the flow of conduction electrons, while the latter can be used to capture the effects of magnetic frustration by building bound molecule-like states of two atoms and confining them in deep energy wells of the lattice. These molecules are not featureless, however: the same nuclear spins that enable their creation also give them an internal structure with different states encoded by red, green and blue colors in the figure.
When an itinerant atom (represented by black spheres in the figure) hops into an energy well, it can rapidly change the state (color) of the bound pair sitting in that well. As itinerant atoms move around the lattice they mix the colors of the local molecules, thus making them appear white and entangled [1]. But at the same time, this process slows down the atoms and makes them appear heavier than they really are, similar to the electrons described earlier. Thus, studying a cold-atom system reveals a peculiar regime in which heavy electrons (modeled by the freely hopping, but heavy, itinerant atoms) can happily exist in a frustrated magnetic system.
Fortunately, the Ye lab will soon be ready to test this complex new theory of conduction in the laboratory with ultracold alkaline-earth atoms in an optical lattice. Stay tuned.––Leonid Isaev, Ana Maria Rey, and Julie Phillips
[1] When particles are entangled, it means that when something happens to one of them, the other responds.
The Physics Frontiers Centers (PFC) program supports university-based centers and institutes where the collective efforts of a larger group of individuals can enable transformational advances in the most promising research areas. The program is designed to foster major breakthroughs at the intellectual frontiers of physics by providing needed resources such as combinations of talents, skills, disciplines, and/or specialized infrastructure, not usually available to individual investigators or small groups, in an environment in which the collective efforts of the larger group can be shown to be seminal to promoting significant progress in the science and the education of students. PFCs also include creative, substantive activities aimed at enhancing education, broadening participation of traditionally underrepresented groups, and outreach to the scientific community and general public.